https://www.marketwatch.com/economy-politics/calendar
Tuesday
# Consumer confidence index for April
Wednesday
# First estimate of Q1 GDP - if it's negative, that means we will have 2 quarters in a row of negative GDP -- the informal definition of a recession -- (the official one, by the NBER National Bureau of Economic Research, usually takes a year after one has started before it to be declared, ditto for recoveries)
Thursday
# The weekly initial unemployment claims
# Personal Income and Consumer Spending for March
Friday
# ISM manufacturing index for April
# Motor vehicle sales for April
On the stock market ... someone posted that we've recently been here before:
In 2018 from peak to trough, the market (S&P 500) declined 19.8% (just 0.2% shy of a bear market)
9/20/18: 2930.75 12/24/18: 2351.10
Whereas we're currently (Friday 4/24/20 close of 2837) down 16.2% from the 3386 all time high on February 19
(Although at the bottom on 3/20/20 it closed at 2305, down 31.9% from the all time high -- which looked like this, on a graph of the S&P 500 all the way back to 1927 or 1928 on a logarithmic scale) :

Details:
https://www.democraticunderground.com/10142452432#post14
I'm fascinated that the dot-com crash and the housing bubble crash look like little notches in a multi-decade history of the S&P 500, and that the 31.9% plunge from the all-time high from Feb 19 - March 20 looks so small. But this is something that
averages a doubling every 9-10 years (even shorter average doubling times on a total return basis with dividends included)
Edited to add: the graph was produced with an interval setting of 1 month (otherwise it wouldn't show the entire 1928-2020 span). So that's why one doesn't see the 3386 2/19/20 close on it, for example -- so actually the 31.9% peak-to-trough plunge is longer than shown on the graph -- but not by much: 3386 would be a very tiny short distance above the 3364 line on the graph. Right click on the graph and choose Open Image in New Tab (or in New Window) to see a bigger version of the graph).
The reason for a logarithmic graph is that a positive-sloping straight line is a constant percent increase -- so for example a climb from 10 to 20 (a doubling) is the same vertical distance on such a graph as a climb from 1000 to 2000 (a doubling).
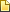 = new reply since forum marked as read
= new reply since forum marked as read
